© S.M.Korotaev

EXPERIMENTAL VERIFICATION OF TRANSACTION OF THE DISSIPATIVE PROCESSES THROUGH THE ACTIVE PROPERTIES OF TIME

S. M. KOROTAEV

Geoelectromagnetic Research Institute, Russian Academy of Science

GEMRI, P.O.box 30, Troitsk, Moscow Region 142190 Russia. E-mail

Abstract

The review of experimental results on verification of Kozyrev¢ s transaction of the dissipative processes through the active time is presented. Such transaction is considered as manifestation of macroscopic nonlocality. The experiment at the modern level of rigor has confirmed existence of nonlocal transaction between various dissipative processes. The most prominent property of this phenomenon is causality in reverse time.

 

1.Introduction

More than 30 years ago N.A. Kozyrev suggested concept of active time, developed in the framework of approach called causal mechanics [1]. Causal mechanics is originated from acceptance of fundamental asymmetry of time. Certainly, time asymmetry leads to violation of the energy conservation. Causal mechanics treated this violation as the energy of time appearance. Therefore, time has, except of the passive property of duration, some active ones (course, density), which involve interaction or, more exactly, transaction between any dissipative processes. Kozyrev performed extensive series of the experiments, which demonstrated unusual properties of this transaction, for example, availability of advanced correlation for non-controlled processes [1-3]. However his results met a contradictory reaction because of weak formalization of the theory and doubt about rigor of the experiments. But recently the basic statements of causal mechanics were strictly formulated [4-7] and the experiments at the modern level of rigor were performed [5,6,8-13]. This paper is devoted to results of these experiments.

The paper is ordered as follows. The next section will shortly review theoretical background and formulate the hypothesis. Section 3 will deal with setting up an experimental problem. Afterwards the experimental setup and data will be described in Sec 4. In Sec.5 description of the most interesting results will be presented. The conclusion will be in Sec. 6.

 

2.Transaction through active time as macroscopic nonlocality

Generalization of Kozyrev¢ s results had shown similarity of the properties of transaction through active time with ones of quantum nonlocality [5-7, 9, 11]. It is generally believed that quantum nonlocality exists only at the micro-level. But during the last years theoretical considerations evolved of nonlocality persistence at the macroscopic limit [13-15], although a viable idea for experimental verification of such conclusion was not suggested (the standard blueprint of the interference experiment at the macro-limit is wittingly unfit). Our idea is to include dissipation by interpretation of quantum nonlocality within Weeler-Feynman action-at-a-distance electrodynamics [17], which we use in modern quantum treatment [18]. This theory considers direct particle field as superposition of retarded and advanced ones. The advanced field is unobservable and manifests only through radiation damping, which is the dissipative process.

But first of all let’s take notice to likeness of axioms of causal mechanics and action-at-a-distance electrodynamics. In the electrodynamics transaction of the charges separated by finite distance d and lapse d t (with zero interval)is postulated. Self-action of the charges is absent. Two from three Kozyrev¢ s axioms [1] assert the same, replacing only terms “charges” by “cause” and “effect” (third axiom postulates time asymmetry), while in Ref.[18] Kozyrev grounded that transaction occurs through zero interval. In Ref.[4] uncertainty of the terms “cause” and “effect” had been removed. Essentiality of the formalism is as follows. For any observables X and Y the independence functions i can be introduced:

(1)

where H denote conditional and unconditional Shannon entropies. For example if Y is single-valued function of X then iYIX = 0, if Y does not depend on X, then iYIX = 1. Roughly saying, the independence functions behave inversely to module of correlation one.

Next, the causality function g is considered:

(2)

It can define that X is cause and Y is effect if g <1. And inversely: Y is cause and X is effect if >1. The case =1 means non-causal relation X and Y (they are related with some common cause).Theoretical and multiple of experimental examples have shown that such formal definition of causality does not contradict its intuitive understanding in obvious situation and can be used in non-obvious ones (e.g.[20-23]). Our definition allows formulating all three Kozyrev¢ s axioms in the form of one:

g < 1 Ю tY > tX , ;

g > 1 Ю tY < tX , ; (3)

g 1 Ю tYtX , .

 

Statement (3) is very natural, but it is axiom of strong or local causality. For nonlocal transaction this statement might be invalid due to advanced correlations of the dissipative processes. Indeed any dissipative process is ultimately related to the radiation and therefore to the radiation damping. As a result it can be shown that advanced field connects the dissipative processes [5-7].

Time asymmetry is expressed as absorption asymmetry. While absorption of retarded field is perfect, absorption of advanced one must be imperfect. Having accepted that total field E is superposition

E = AE ret + BE adv , (4)

Where A and B are constants and having denoted efficiency of absorption of retarded field by a (a=1 corresponds to perfect absorption, a=0 – to absence of absorption), advanced one by b, it is easy to obtain [18], that

A = , B = , (5)

Substitution to Eq. (4) A=1, B=0 corresponds to really observing situation, that is compatible with Eq. (5) only if a=1, 0 £ b < 1. It should be stressed wide a priori arbitrariness in value b, which may be close as to unit so to zero. Therefore the screening properties of the matter must be in one degree or another attenuated. The fact itself of imperfect absorption of the advanced field means a possibility of its separate detection.

From the operational consideration it is possible to formulate the following equation:

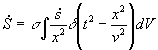, (6)

~ , ,

where is the entropy production in a probe process (that is detector), is entropy production in the sources, s is cross-section of transaction. The d -function shows that transaction progresses with symmetrical retardation and advancement. In particular, if the transaction occurs through a medium by diffusion, then values of resulting retardation and advancement are large.

 

3. Setting up the experimental problem

The experiment task is to detect the entropy change of the environment according to Eq (6) under condition that all known classical local interactions are suppressed. Two types of detectors had been chosen by the criterion of maximal efficiency of a probe process: the first was based on the variations of self-potentials of weakly polarized electrodes in an electrolyte, the second – on the variations of dark current of the photomultiplier Consider briefly, for example, theory of the electrode detector.

Self-consistent solution for the potential u in the liquid phase is [24]:

(7)

where q is charge of the main ion of the liquid phase, x is dimensionless length (x = 1 corresponds to half of the distance between the electrodes), z is full (electrokinetic) potential. The entropy S can bee expressed in term of the normalized potential j :

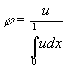, (8)

, (9)

Substituting Eq. (7) to Eq. (8) and (9), one can obtain the expression for the entropy:

S » ln6-2ln(arccosexp w), (10)

Where

.

Therefore, the entropy production is:

(11)

Prefactor of w is always positive, therefore from Eq. (10) and (11) it follows that S and change in opposite phase. So far as variations of z are small in comparison with the averaged value, one can linearize Eq. (11) and obtain final simple expression:

(12)

All known local factors influencing on z : temperature, pressure, chemism, illumination, electric field, concentration and movement of the electrolyte must be excluded. In fact, only difference U= z 1 - z 2 of pair of the electrodes can be measured. Except external screening, influence of mentioned above noise-forming factors might be minimized by measuring U at minimal electrode separation. In this case variable U is:

, (13)

where z c are constants, g is efficiency of the detector, the averaged measure of which is the variability coefficient.

For the photomultiplier detector analogue of U is the work function. Noise-forming factors to be excluded are: temperature, electric and magnetic field, illumination, moisture and feed voltage instability.

It is known that quantum nonlocality violates strong causality and persists weak one [17]. It means, that if a source-process is noncontrolled, we can observe both retarded and advanced correlations. But if an observer initiates a source-process, only retarded correlations are possible. That is why the most interesting source-process are large-scale natural ones. The experiment described below was devoted to study detectors reaction on various geophysical and astrophysical processes. The experiments with controlled lab artificial source-processes had also been conducted, though they had, of course, demonstrated only retarded correlations [12,13].

 

4. Experimental setup and data.

The Geoelectromagnetic Research Institute (GEMRI) experimental setup included two types of detectors and apparatus for accompanying measurements.

The detector based on weakly polarized electrodes was constructed as follows. As the electrodes marine geophysical C-Mn ones were chosen. The electrodes were positioned in the glass vessel with the NaCl water solution; separation between contact windows measured 1.5 cm. The vessel was rigidly encapsulated so that evaporation as well as atmospheric pressure variations were fully eliminated. The vessel was positioned in the dewar, covered on the outside by the additional layers of heat insulation. For residual temperature variations control the sensor of temperature (allowing to measure it continuously accurate to 0.001 K) was positioned between internal wall of the dewar and the electrode vessel. Thus influence of all noise-forming factors, except temperature, was eliminated. Influence variation of the last was minimized and controlled. The quantity U was measured continuously accurate to 0.5 mV.

The second type detector was constructed on the base of photomultiplier with the Cb-Cs cathode of small area. The photomultiplier was positioned in the similar dewar with the temperature sensor and the additional external electric field screen. Possible magnetic field influence was controlled by quantum modulus magnetometer accurate to 0.01 g. The dark current I was measured continuously accurate to 0.05 nA. Magnetic field measurement served also as indicator of the most important for quantitative interpretation geophysical process – dissipation of ionospheric electric current.

Lastly, the overall air temperature in the lab was recorded continuously accurate to 0.1 K. Thus measurements on the setup included 2 major channels and 4 satellite ones. Accidentally during the part of period of the experiment with the setup described above and independently, similar measurements of Ag-AgCl marine electrodes self-potentials in other purposes were conducted by V.I. Nalivayko, kindly presented us his data. His setup did not provide measurements of the noise-forming factors and protection from them. Nonetheless, if a signal associated with the geophysical processes in U variations is sufficiently strong then, taking into account relatively small distance between the labs (300 m), it would have hoped on correlation of data.

Moreover, during the part of period of the GEMRI experiment, the experiment with similar aim, but with another technical realization was performed by A.N. Morozov at Center of Applied Physics (CAP) Moscow State Technical University. The CAP setup [25] included detector measuring fluctuation of conductivity (corresponding to fluctuation of ions mobility) in the electrolyte cell. These fluctuations were measured as high-frequency (5...15 kHz) voltage fluctuation on the cell under applied stabilized low-frequency (3 Hz) current. The steps taken to screening of detector against local influences were comparable with those of the GEMRI setup. Internal temperature of the detector was controlled as well as in the GEMRI detectors. External atmospherical temperature was continuously measured as index of the synoptic activity.

GEMRI setup measurements carried out in continuous regime during 366 days from 1996, December 10 to 1997, December 11. A data set included signal of the electrode detector U, its internal temperature TU, signal of the dark current detector I, its internal temperature TI, the external lab temperature Te and modulus of geomagnetic field F. During the first month data sampling was chosen 5m, then - 30m, and in the last two days - 1m. Simultaneous data spaced at 300m the setup of N.A. Nalivayko Ur and spaced at 40 km the CAP setup b (averaged over 5m intervals disperse of the voltage fluctuations on the electrolyte cell), were invoked for joint interpretation. The temperature of air in Moscow, the atmospheric pressure in Troitsk and standard international data on the geomagnetic and solar activity (radio wave flux at 9 standard frequencies within range 245 … 154000 MHz) were taken to study the large-scale processes. In addition, data on cosmic ray counting rates from the IZMIRAN neutron monitor, situated near (at distance 100 m) the GEMRI setup, were invoked as one more reasonable local factor of influence on the detectors.

Data were processed by the methods of causal, correlation, regression and spectral analysis.

 

5. Results

5.1. RELATION OF THE SIGNALS OF DIFFERENT DETECTORS.

Thus we had long-term measurements with 4 detectors of 3 types. Their signals proved to be rather high correlated. Mathematical exclusion of single possible common local factor not completely suppressed by screening, namely internal temperature leads to ase correlation increasing. Therefore there is not any local common cause of the signals. Level of correlation proved to be independent on type of detectors and only slightly dependent on their separation. Namely, correlation between nearby detectors rUI = 0.78 0.01, spaced at 300 m rUUr = 0.74 0.01 and spaced at 40 km rUb =0.720.01. Such correlation can be explained by some large-scale common causes (geophysical processes), but their influence cannot be local.

Due to that correlation we shall consider in the following subsection mainly results with the electrode detector U.

5.2. RELATION OF THE DETECTOR SIGNAL WITH THE INTERNAL AND EXTERNAL TEMPERATURE.

First of all, the environment temperature variations lead to its entropy changes. The problem is complicated by trivial local influence of small residual variations of internal temperature on the probe process, but we know that such influence can be only retarded.

Due to passive thermostating, dispersion of the internal temperature TU in the dewar of the detector U is very small (it is decreased on two orders relative to one of the external lab temperature Te). Indeed, there is small correlation pike =-0.33 ±0.02 (corresponding to the normal negative temperature coefficient of the electrodes -141±9 mV/K [26]), which is accompanying by minimum of the independence function at the time shift t = -20.4h (negative sign of t corresponds to retardation U relative to TU). But at the positive time shift t=12.8h (where correlation must be classically damped out) there is unusual great correlation pike =0.87±0.01 (anomaly positive sign) which is accompanying by minimum of .

Turn now to analysis of connection U with the external (lab) temperature Te. As there are no heat sources inside of the dewar, where TU is measured, then local connection of the potential variations with the temperature works along the causal chain Te®TU®U. It imposes the restrictions on independences [21]:

, . (14)

Violation of Ineq. (14) (which are macroscopic analogue of Bell’s inequalities) is sufficient evidence of nonlocality of transaction Te and U. It has turned out that i(t) has 3 almost symmetrical minima at t=0 and ± 27.0h. It corresponds qualitatively to results of known Kozyrev’s astrophysical experiment [2,3]. Asymmetry amounts to stronger advanced signal as compared to retarded one: at t=-27.0h at t=0 at t=27.0h . Therewith independences of TU and Te have only single normal minimum: at t = -11.5h , i.e. Te®TU. Substituting these and mentioned above values of independences TU and U, we concluded that there are two channels of connection Te and U: classical local retarded and unusual nonlocal advanced. For the former at t<0 left Ineq. (14) is asserted, for the last at t>0 right Ineq. (14) is violated.

Availability of the synchronous channel of connection Te with U is explained by interference of the retarded and advanced signals [17]. But symmetry by t for Te, U with asymmetry for TU, U calls for analysis.

The space-time of transaction of Te, TU, U is schematized in Fig. 1. t ret and tadv denote times of information passage along the retarded and advanced channel respectively, other notations are clear from the figure. Te and TU both are connected with U by pairs of the symmetrical retarded and advanced channels, while Te connected with TU only by retarded one, it follows from geometry of this scheme that dadv=tadv(U|Te)-tadv(U|TU)= tadv(U|Te)-[tret(U|TU)- tret(TU/Te)]. But tret(TU/Te)= tret(U/Te)-tret(U/TU)=dtret. Therefore dadv =2dret. Substituting mentioned above values t, one can be content with dadv =2dret accurate to 7%. Therefore the diagram of Fig. 1 quantitatively explains the positions of all pikes of independence functions.

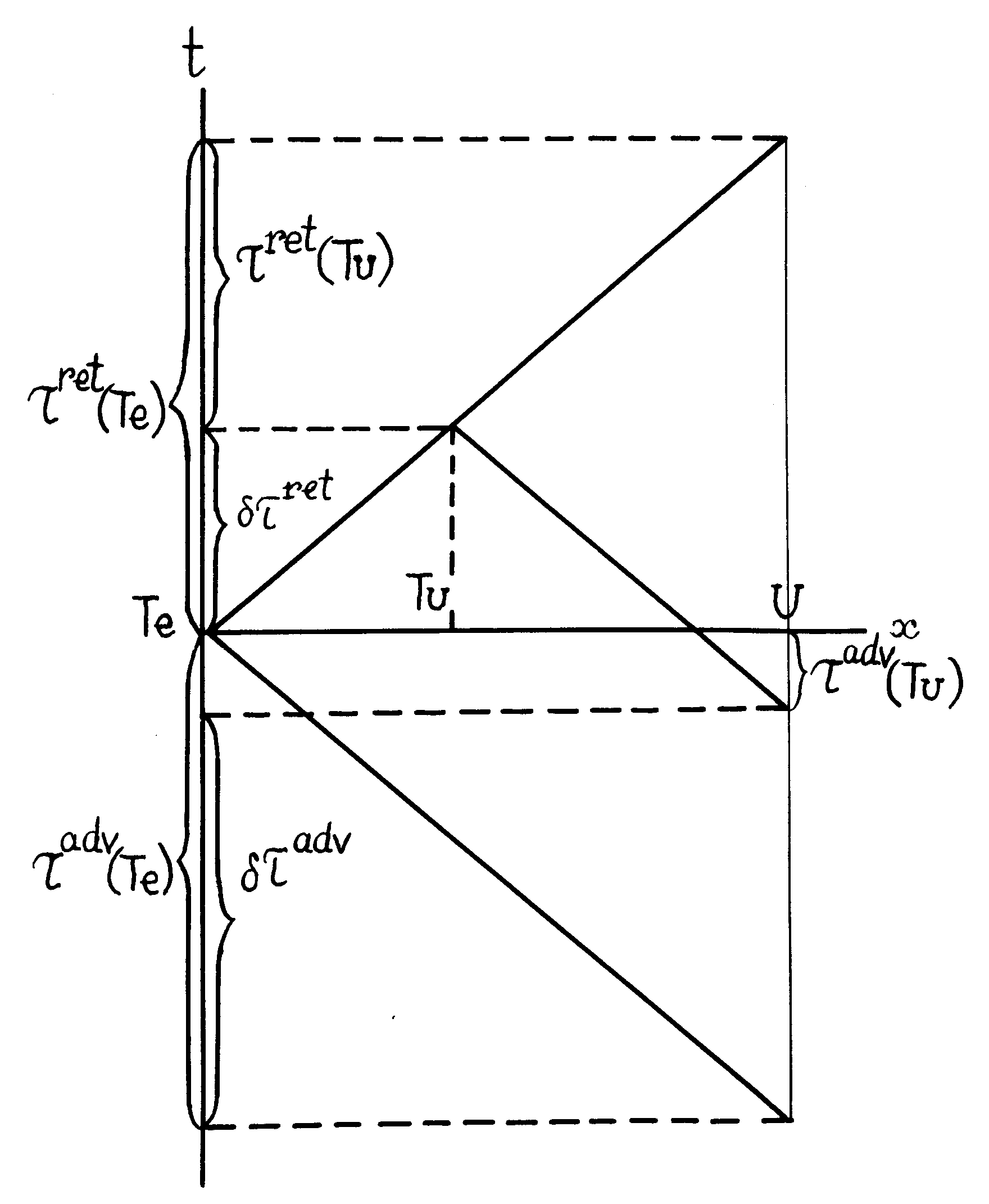
Figure 1. Diagram of transaction of the external (lab) temperature Te, internal temperature TU and self potential difference of the electrodes U. Scales of distance x and time t are arbitrary. tret and tadv are respectively retardation and advancement of the temperatures (TU or Te) relative to U. dret and dadv are differences of respective tret and tadv.

5.3. RELATION OF THE DETECTOR SIGNAL WITH THE SYNOPTIC ACTIVITY.

Consider now the variations of the atmospheric temperature Ta as an index of the synoptic activity. Taking into account passive thermostating, the local causal connection Ta®Te®TU®U must lead to weak correlation U with Ta at very long (many-days) retardation. Following usual geophysical practice of study of the large-scale processes, for exception of a possible influence of the small-scale inhomogeneities compare measurements U and Ta at remote sites. Under a typical horizontal temperature scale (a few hundred km), distance between the GEMRI and CAP setups (40km) is quite optimal. That is why the measurements of Ta near CAP setup have been taken for comparison with U.

The most important feature of U,Ta dependence is a dramatic exceeding of correlation at advancement U relatively Ta (t>0) above weak (less than 0.4) retarded correlation (t<0). Next there are five maxima at t equal to -25, -13, 0, 13, 28 days. Symmetry relatively t =0 is exactly analogously described above relation U with Te. The greatest correlation is at t =13d: =0.725±0.005, The causal analysis has shown corresponding minima of the independence function (at t=13d =0.72±0.01, , that is Ta ® U). Thus there is statistical reliable advanced connection of U to Ta.

More appropriate synoptic activity index is the atmospheric pressure, which scale a few thousand km . In Fig. 2 an example of causal analysis of the electrode detector signal and pressure is shown. At advancement 69 days ( rUP = - 0.78 ± 0.02 ) there is a deep independence functions minimum and high causality function pike (. The synoptic activity is a cause of the detector signal, but the progress in reverse time!

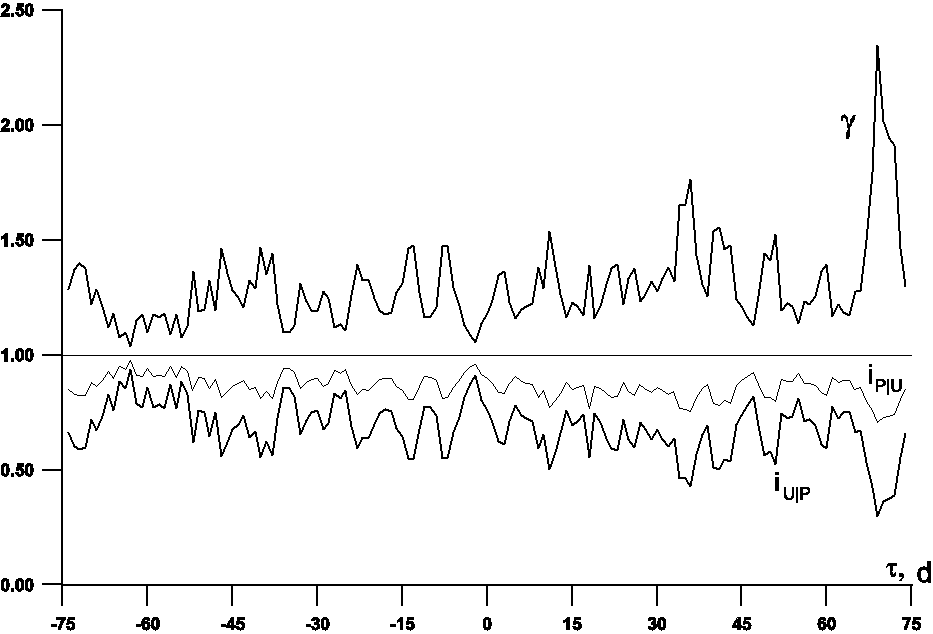
Figure 2. Independence i and causality g functions of the electrode detector signal U (March – April, 1997) and the atmospheric pressure P. t is time shift of P relative to U in days.

That result is independent on type of detector. In Fig. 3 the same example with the photomultiplier detector is shown. The picture is alike and advancement is almost the same, it equals 73 days (rIP = - 0.86 ± 0.01).

Figure 3. Independence i and causality g functions of the photomultiplier detector signal I (March – April, 1997) and the atmospheric pressure P. t is time shift of P relative to I in days.

It is even possible to give the simplest forecast. In Fig. 4 fore the same example time variation of pressure (passing a cyclone) and preceding on 73 days variation of the dark current are shown.

Figure 4. The variation of the detector signal I forecasting variation of atmospheric pressure P with advancement 73 days. The origin of time corresponds to March 24, 1997.

5.4. RELATION OF THE DETECTOR SIGNAL WITH THE GEOMAGNETIC

ACTIVITY.

It is beyond reason to consider U dependent on magnetic field F by any way. Therefore detection of relation of the potential with the Earth magnetic field variations would be a good test for the hypothesis (6), as these variations could be easily related with electric current dissipation in the source (ionosphere). Special experiments on influence on the detector of U by artificial magnetic field (up to 100A/m) in frequency range from 0 to 1 Hz had confirmed absence of any reaction of U within sensitivity of the apparatus.

Analysis of long time series has shown existence of stable correlation rUF=-0.56 ±0.01 only with advancement U relative to F (t=48.0h) . At this t there is minimum iF|U=0.79. Thus relation U and F is statistically reliable, but both from prior reasons and from advancement U relative to F it can not be result of a direct influence F on U. Therefore F is indicator of some process interacting with U.

The spectral analysis in the range of maximal geomagnetic activity (10-6…10-5Hz) has shown that frequency dependence of amplitude ratio U/F is approximated by formula

(15)

In terms of spectral densities Eq. (15) turns into

, (16)

where , f is frequency. Eq. (16) describes flicker-noise, although we cannot localize the noising resistivity.

Whereas U(f)/F(f) depends on frequency f, it has proved that U(f)/F2(f) does not depend on f: U(f)/F2(f)=(1.7±0.2)·10-5W m2/A. It is the most important result pointing to relation of U with the source entropy production.

For proof consider application of Eq.(6) to the concrete case. Magnetic field F is related with electric currents in the source – ionosphere, and also with induced currents in the Earth. For simplicity of the problem, neglect by the last and consider entropy production only in the source of F. It is easily to express the density of entropy production through electric field E(f) (which in turn through impedance Z(f) is related with F(f)), resistivity r and medium temperature T. r and Z(f) consider for simplicity as scalar. Then:

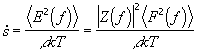. (17)

Combining Eq. (6), (12), (13) and (17) we obtain:

. (18)

Eq. (18) can be simplified using the known properties of electromagnetic field of the ionospheric source [27]. First, the field F is well approximated by the plane wave, therefore it is possible to factor out the F2 from the integral. Second, using quasi-steady-state approximation of the plane wave impedance, substitute | Z(f)|2 =2p fm0 r in Eq.(18). As a result we have:

(19)

Thus the experimental fact U(f)/F2(f)=const is explained within the Eq. (6).

It is of interest to estimate the constant s from observations. As on this stage only order of s is of interest, we can simplify Eq. (19), supposing, that similarly to an ordinary electromagnetic field, it is possible to employ the plane wave approximation. Then, instead of Eq.(19), we have

, (20)

where h is thickness of the dynamo-layer. For estimation of s accept corresponding to the detector parameters: TU=3·102K, q=1.6·10-19 A·s, g=6·10-2, and known typical ionospheric parameters: T = 1U3K, h=5·104m. Then with mentioned above value U(f)/F2(f) we obtain from Eq.(20) s=2·10-21m2. The experimental and theoretical estimations are of the same order.

To stress large scale of the process we can go from magnetic field measured by setup’s magnetometer to indices of global geomagnetic activity. The most appropriate one proved to be Dst – index (which is calculated by data equatorial geomagnetic observatories). The advancement of the detector signal relative to global activity turned about month. After appropriate filtration we also can give forecast of the geomagnetic activity. In Fig. 5 electrodes self-potential difference U forecasts the Dst – index of geomagnetic activity with advancement 33 days.

Figure 5. The variation of the detector signal U forecasting variation of the Dst-index of geomagnetic activity with advancement 33 days. Time origin corresponds to January 11, 1997.

5.5. RELATION OF THE DETECTOR SIGNAL WITH SOLAR ACTIVITY.

The spectral analysis has shown similarity of the signal and solar activity indices spectra. In particular there is maximum at the synodic period of solar rotation (27d) with amplitude of U about mill volt, and I about nanoamper.

Time domain analysis gives more interesting information. Since time averaged-terrestrial data always demonstrate stronger dependence our, data were processed with daily and monthly averaging. As the solar activity indices the solar radio wave flux at 9 standard frequencies within range 245…15400 MHz were taken.

Analysis of daily averaged data has shown that both maximum of correlation and minimum of independence functions correspond to frequency 1425 MHz. This frequency corresponds radiation from the level of lower corona-upper chromosphere that is just from the level of maximal dissipation.

One can suggest the cosmic ray flux as possible mechanism of influence of the solar activity on detectors. Checking has shown that correlation of the detector signals with cosmic ray counting is statistically insignificant.

Maximum of dependence U on R (corresponding to min i U|R ) is at large advancement U relative to R. For the optimal frequency 1415 MHz (and max g = iR|U / iU|R = 1.41 ± 0.01) is at t = 39d.

Monthly averaged data have demonstrated this advancement connection even more brightly (though the causal analysis by depleted series is impossible). In Fig 6 such data of U and R shifted on 1 month are presented. The strong advanced correlation is evident At t = 1M rUR = 0.76 ± 0.08.

Figure 6. Time variation of monthly smoothed-out detector signal U forecasting variation of monthly smoothed-out solar radio wave flux R at frequency 1415 MHz. Time origin corresponds to December, 1996. The time interval spans the beginning of a regular solar activity cycle.

6. Conclusion

The experiment on the modern level of rigor has confirmed existence of Kozyrev¢ s transaction of the dissipative processes through active time, which can be understood now as manifestation of macroscopic nonlocality. The most prominent property of this phenomenon is transaction in reverse time. It gives the possibility of observation of noncontrolled future. Of course, our theoretical approach was essentially heuristic. Therefore development of the theory at crossing of quantum nonlocality, action-a-distance electrodynamics and causal mechanics is burning.

 

Acknowledgements

This work was supported by RFBR and Moscow Region Government (grants 01-05-97015 and 02-05-64006). The author thanks J.V. Gorohov and V.O.Serdyuk for participation in data processing.

 

References

  1. Kozyrev,N.A.( 1971). On the Possibility of Experimental Instigation of the Properties of time, in J. Zeman (ed.), Time is Science and Philosophy, Academia, Prague, pp.111-132.

  2. Kozyrev, N.A. and Nasonov, V.V., (1978). Method of determination of trigonometric Parallaxes on the base of difference Between Actual and Visible Position of the Stars, in A.A.Efimov (ed.), Astrometry and Heavenly Mechanics , VAGO Press, Moscow – Leningrad, pp 168-179.

  3. Kozyrev, N.A. and Nasonov, V.V., (1980). On some Properties of time detected by Astronomical Observations, in A.A.Efimov (ed.), Manifestation of Cosmic Factors on the Earth and Stars, VAGO Press, Moscow-Leningrad, pp.76-84.

  4. Korotaev, S.M., (1993). Formal definition of causality and Kozyrev’s axioms, Galilean Electrodynamics, 4, 86-89.

  5. Korotaev, S.M., Serdyuk, V.O., Sorokin, M.O. and Abramov, J.M., (1998). Experimental study of macroscopic nonlocal interaction of the dissipative processes, Physical Thought of Russia, 2, 1-17.

  6. Korotaev, S.M., Serdyuk, V.O., Sorokin, M.O. and Abramov, J.M., (1999). Experimental study of macroscopic nonlocality, Science & Technology in Russia,1, 16-19.

  7. Korotaev, S.M., (2000). The force of time, Galilean Electrodynamics, 11, 29-33.

  8. Dvoruk, S.K., Korotaev, S.M., Morozov, A.N., Nazolin, A.L., Serdyuk, V.O., Solovyov, A.V., Sorokin, M.O., Tabalin, S.E. and Shishkin, G.V., (1998). Experimental studies of the irreversible processes in the electrolytes, Applied Mechanics and Machine-Building Technologies, 1, 61-66.

  9. Korotaev, S.M., Sorokin, M.O. Serdyuk, V.O. and Abramov, J.M. (1999). Geophysical manifestation of interaction of the processes through the active properties of time, Physics and Chemistry of the Earth. 24. 735-740.

  10. Korotaev, S. M., Serdyuk, V.O. and Sorokin, M.O. (2000). Experimental verification of Kozyrev’s Interaction of Natural Processes, Galilean Electrodynamics, 11, 23- 28.

  11. Korotaev, S.M., Serdyuk, V.O. and Sorokin, M.O. (2000). Effect of macroscopic nonlocality on geomagnetic and solar-ionospheric processes, Geomagnetism and Aeronomy, 40, 323-330.

  12. Korotaev, S.M., Serdyuk, V.O, Sorokin, M.O. and Machinin, V.A. (2000). Experimental study of nonlocality of the controlled dissipative processes, Physical Thought of Russia, 3, 20-26.

  13. Korotaev, S.M., Serdyuk, V.O, Sorokin, M.O. and Machinin, V.A. (2000). Experimental study of effect of artificially exited dissipative processes, Herald of DGGGMS RAS, 3, 100-107.

  14. Mermin, N.D., (1990). Extreme quantum entanglement in a superposition of macroscopically distinct states. Phys. Rev. Lett., 65, 1838-1840.

  15. Roy, S.M. and Singh, V., (1991). Tests of signal and Einstein-Bell locality for multiparticle systems, Phys.Rev. Lett., 67, 2761-2764.

  16. Home, D. and Majumdar, A.S. (1995). Incompatibility between quantum mechanics and classical realism in the strong macroscopic Limit, Phys. Rev. A., 52, 4959-4962.

  17. Cramer, J.S., (1980). Generalized absorber theory and the Einstein-Podolsky-Rosen paradox, Phys.Rev D, 22, 362-376.

  18. Hoyle, F. and Narlikar, J.V., (1995). Cosmology and action-at-a-distance electrodynamics, Rev. Mod. Phys., 67, 113-156.

  19. Kozyrev, N.A., (1980). Astronomical proofs of reality of 4D Minkowski geometry, in A.A. Efimov (ed.), Manifestation of Cosmic Factors on the Earth and Stars, VAGO Press, Moscow-Leningrad, pp 85-93.

  20. Korotaev, S.M., (1992). On the possibility of causal analysis of the geophysical processes, Geomagnetism and Aeronomy, 32, 27-33.

  21. Korotaev, S.M., Shabelyansky, S.V. and Serdyuk, V.O.,(1992).Generalized causal analysis and its employment for study of electromagnetic field in the ocean. Izvestia Phys. of the Solid Earth, 6, 77-86.
  22. Korotaev, S.M., Hachay, O.A. and Shabelyansky, S.V., (1993).causal analysis of the process of horizontal informational diffusion of electromagnetic field in the ocean, Geomagnetism and Aeronomy,33, 128-133.
  23. Korotaev, S.M., (1995). Role of different definitions of the entropy and its employment to electromagnetic induction in the sea currents, Geomagnetism and Aeronomy, 35, 116-125.
  24. Korotaev, S.M., (1979). Filtration electromagnetic field of the submarine springs, Izvestia Phys. of the Solid Earth, 8, 91-95.
  25. Morozov, A.N., (1997) Irreversible Processes and Brownian Motion, BMSTU-Press, Moscow.
  26. Zimin, E.F. and Bogorodsky, M.M., (1996). Modern methods and tools for study of the geoelectric fields, Izvestia Physics of the Solid Earth,10, 31-40.
  27. Rokityansky, I.I., (1982). Geoelectromagnetic Investigation of the Earth’s Crust and Mantle, Springer-Verlag, Berlin.